The proof of the above proposition is a direct verification based on
definitions.
The study of closeness of the partial minimum is based on the following
observation.
Suppose the level set
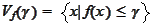 is nonempty for some
is nonempty for some
 .
Let
.
Let
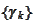 be a sequence such that
be a sequence such that
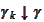 .
Then
.
Then
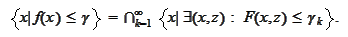 The set
The set
 is closed if
is closed if
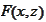 is closed. The set
is closed. The set
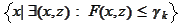 is a projection of
is a projection of
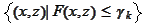 .
Its closeness may be studied by means of the proposition
(
Preservation of closeness
result
). The intersection preserves the closeness. Hence, we arrive to the
following proposition.
.
Its closeness may be studied by means of the proposition
(
Preservation of closeness
result
). The intersection preserves the closeness. Hence, we arrive to the
following proposition.
|