he reference for most of material here is
[Evans]
.
The main result of this chapter is negative: even though there is a Lagrangian
for the heat equation nevertheless the Hamiltonian technique does not apply.
However, the method of characteristics is separately useful.
We are considering a PDE of the
form
|
|
(First order PDE)
|
where the
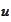is an unknown function
,
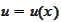the variables
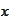are from
,
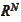the
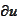refers to the vector of derivatives
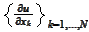and the function
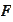is smooth. We will use the following notation for the function
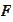's
arguments:
|
