e would like to calculate the
expression
 Observe that
Observe that
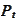 is not a martingale.
Indeed,
is not a martingale.
Indeed,
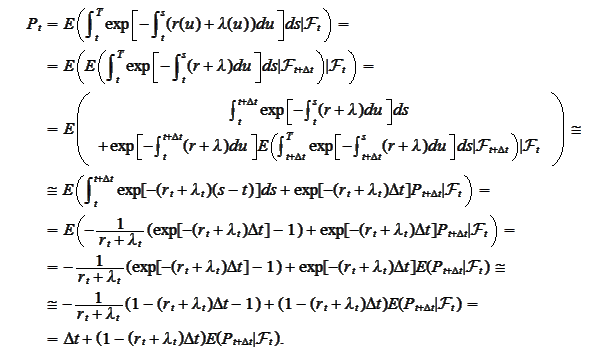 Hence,
Hence,
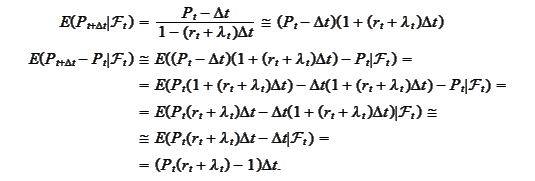 We conclude
that
We conclude
that
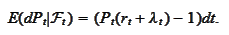 Therefore,
Therefore,
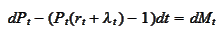 for some martingale
for some martingale
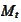 .
We want to replicate the
.
We want to replicate the
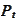 with some function of state variables
with some function of state variables
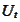 .
If successful, such function should
satisfy
.
If successful, such function should
satisfy
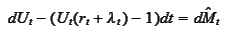 for some martingale
for some martingale
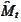 .
If both martingales also coincide at final time
.
If both martingales also coincide at final time
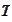 then we will have the
equality:
then we will have the
equality:
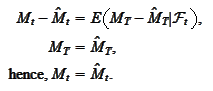
We will seek for
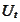 in the
form
in the
form
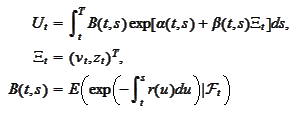 where the
where the
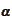 and
and
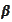 are some deterministic functions.
Hence,
are some deterministic functions.
Hence,
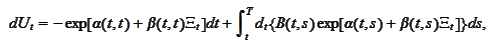 We introduce
notation
We introduce
notation
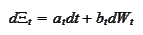 with
with
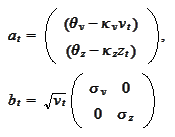 then
then
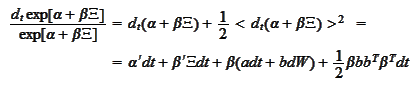 hence we calculate the drift part as
hence we calculate the drift part as
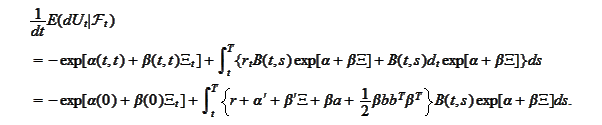 We want the above expression to be
We want the above expression to be
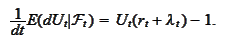 Hence, it suffices to
have
Hence, it suffices to
have
 where
where
 Consequently,
Consequently,
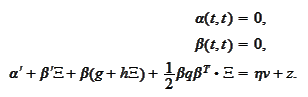 We separate the
coordinates
We separate the
coordinates
 We substitute the expressions for the
We substitute the expressions for the
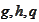 .
The equations resolve
to
.
The equations resolve
to
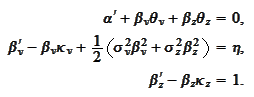 These coincide with the equations (17) in the article because (in article
[CarrWu2006a]
's
notation)
These coincide with the equations (17) in the article because (in article
[CarrWu2006a]
's
notation)
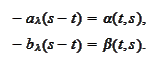
|