e would like to derive conditions for the vector
to be unique.
The defining relationship is (we omit the upper index
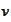on
)
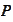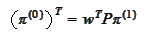or
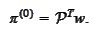To have uniqueness we must make sure that
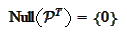where the
Null
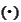is the notation for null set. In
general,
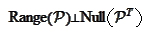hence, it is enough to show that
has the full range.
We introduce the notion of acceptable completeness.
Definition
The market is acceptably complete iff any payoff
may be hedged with existing assets so that the remaining risk is
acceptable:
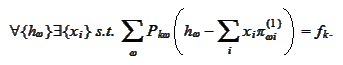
In other
words
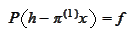or
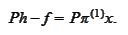Hence, it is enough to make sure that
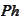spans the full space. This means that the space of cash flows is at least as
rich as the structure of the test measures.
Summary
Assuming that
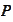has the maximal rank, the pricing vector is unique iff the market is
acceptably complete.
|
