his section follows
[Avellaneda1995]
.
Let
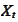be a traded asset and the only state variable
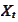is given by the
SDE
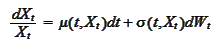under the risk neutral measure. We are considering a situation when the
analytical form of the function
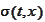is not known. However, we do assume
that
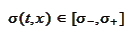for all values of arguments. Following the conclusion
(
Incomplete market ask
) we are
seeking
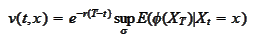for some final payoff function
.
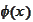We introduce the
notation
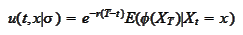for the value of the derivative
dependent on the particular assumption
about the volatility. For any
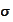and all
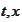we
have
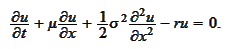The supremum is approached by some sequence
.
Under sufficient regularity restrictions on the class of
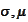we pass the above PDE to the
limit:
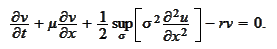Clearly, the supremum is achieved
if
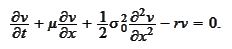Since the
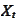is the only state variable, the delta hedging is performed in the regular way
using the
.
|
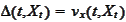