his section follows the article
[CarrMadan2000]
.
We consider economy that has two time moments and a finite space of future
realizations of the world (finite number of "states"). The present is the time
moment 0. The future is the time moment 1. The states are indexed by
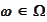 .
There is no cost of borrowing or there is a riskless asset and we measure
prices in units of such asset. We introduce several points of view on
development of the market, indexed by
.
There is no cost of borrowing or there is a riskless asset and we measure
prices in units of such asset. We introduce several points of view on
development of the market, indexed by
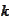 .
We use the following notation:
.
We use the following notation:

|
|
(Finite space variable incomplete market)
|
The position with the
property
 is "0-cost portfolio" or "opportunity". An "acceptable opportunity" is a
vector
is "0-cost portfolio" or "opportunity". An "acceptable opportunity" is a
vector
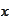 such that
such that
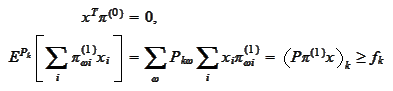 for some vector of "floors"
for some vector of "floors"
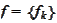 .
.
We divide our models into two categories. Valuation category
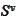 consists of regular models (probability measures) representing reasonable
expectations about development of the market. In order for an opportunity
consists of regular models (probability measures) representing reasonable
expectations about development of the market. In order for an opportunity
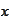 to be acceptable we require that
to be acceptable we require that
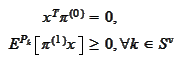 for such models. The measures
for such models. The measures
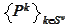 are called "valuation measures". The other category is stress models
are called "valuation measures". The other category is stress models
 .
Stress models represent extreme point of view about market behavior. These are
introduced to exclude infinite risk taking along opportunities accepted by
valuation measures. It is also a representation of common risk management
practices. We require that
.
Stress models represent extreme point of view about market behavior. These are
introduced to exclude infinite risk taking along opportunities accepted by
valuation measures. It is also a representation of common risk management
practices. We require that
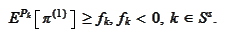
|
|
(Stress tests)
|
There is no point in introducing a stress measure for a situation that is not
considered possible under any reasonable assumptions. Conversely, if we
believe that certain situation requires a stress measure then it better have
non zero probability under some reasonable model. Hence, we require that the
union of supports of all stress measures would be included in the union of
supports of all valuation
measures:
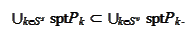 Valuation measures may be obtained via empirical research, hedging argument or
via utility function argument. For example,
Valuation measures may be obtained via empirical research, hedging argument or
via utility function argument. For example,
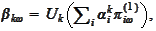
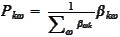 where, as usual,
where, as usual,
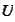 stand for a utility function (with opinion index
stand for a utility function (with opinion index
 )
and
)
and
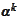 is a position vector of the market participant, responsible for the opinion.
is a position vector of the market participant, responsible for the opinion.
A strictly acceptable opportunity is an acceptable opportunity
s.t.
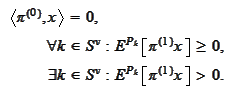 Such opportunity should be taken. Hence, one may assume that market would
reach a state when there are no strictly acceptable opportunities. The
assumption of "no acceptable opportunity" is stronger then the assumption of
"no arbitrage". Hence, the existence of some (not necessarily unique) risk
neutral measure is given under the "no strictly acceptable opportunity"
assumption. There is however a stronger
consequence:
Such opportunity should be taken. Hence, one may assume that market would
reach a state when there are no strictly acceptable opportunities. The
assumption of "no acceptable opportunity" is stronger then the assumption of
"no arbitrage". Hence, the existence of some (not necessarily unique) risk
neutral measure is given under the "no strictly acceptable opportunity"
assumption. There is however a stronger
consequence:
 We call such
We call such
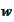 a "pricing vector".
a "pricing vector".
The uniqueness statement of the theory is connected with the notion of
acceptable completeness of the market. The market is acceptably complete if
any time-1 payoff could be hedged so that the residue, after applying of the
hedge, is an acceptable opportunity. Under condition that there are more
assets (index
 )
than models (index
)
than models (index
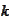 ),
the market is acceptably complete if and only if the vector
),
the market is acceptably complete if and only if the vector
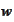 is unique (see the following sections for justification).
is unique (see the following sections for justification).
Bid-ask spread is defined as a minimal price of a hedge, transforming an
exposure into an acceptable opportunity. Note that under such setting the
bid-ask spread is dependent on the current position of the market participant.
In particular, a market maker is justified in modifying a bid-ask spread after
each trade.
|