he expression
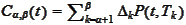located in the denominator of the (
Swap rate
) is a
linear combination of prices of traded instruments. It is always positive.
According to formula (
Suitable numeraire
),
may be taken as a numeraire. The probability measure associated with the
numeraire
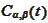is called the "swap measure". The numerator of the formula
(
Swap rate
) is also a price of a traded instrument.
Therefore, the quantity
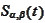is a martingale under the swap measure.
One may justify use of Black-Scholes formula for pricing of swaption using the
notion of swap measure. Indeed, price of swaption is given
by
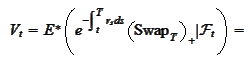
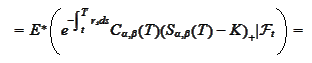
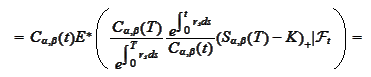
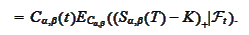We arrived to the Black-Scholes situation if
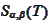is assumed to be log-normal.
|
