n this section we prove that if the
function
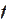of the problem (
Elliptic Dirichlet
problem
) is smooth and the boundary
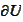is
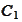then the solution
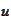is smooth. The general strategy is to start from the weak
problem
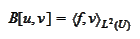and substitute
.
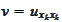Then we would integrate by parts in the
and arrive to the situation of the
form
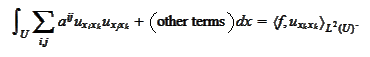Where the "other terms" contain lower derivatives of
.
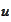We would use the uniform positiveness of the matrix
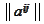to conclude
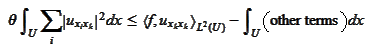for some
.
Consequently, we estimate the right hand side from the above using standard
means (such as the formula (
Cauchy
inequality with epsilon
)) and conclude
.
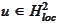The uniform positiveness of the matrix
is a pointwise property. Hence, we cannot use weak derivatives and we are not
given an apriori existence of
.
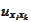Therefore, we will be using finite difference approximations and passing it to
the limit. Such operation requires some preliminaries.
|
