Quantitative Analysis
Parallel Processing
Numerical Analysis
C++ Multithreading
Python for Excel
Python Utilities
Services
Author
Printable PDF file
I. Basic math.
II. Pricing and Hedging.
III. Explicit techniques.
IV. Data Analysis.
V. Implementation tools.
VI. Basic Math II.
1. Real Variable.
2. Laws of large numbers.
3. Characteristic function.
4. Central limit theorem (CLT) II.
A. Lyapunov central limit theorem.
B. Lindeberg-Feller central limit theorem.
5. Random walk.
6. Conditional probability II.
7. Martingales and stopping times.
8. Markov process.
9. Levy process.
10. Weak derivative. Fundamental solution. Calculus of distributions.
11. Functional Analysis.
12. Fourier analysis.
13. Sobolev spaces.
14. Elliptic PDE.
15. Parabolic PDE.
VII. Implementation tools II.
VIII. Bibliography
Notation. Index. Contents.

Central limit theorem (CLT) II.


he CLT was introduced on elementary level in the section ( Central limit theorem ). The calculation of that section has restrictive assumptions and the result lacks generality. Here we consider the issue with greater precision.

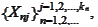
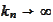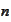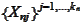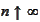Let MATH MATH be a family of r.v. We assume that for each fixed $n$ the family MATH is independent. This chapter studies limiting distributions of MATH as $n\uparrow\infty$ .

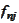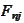We denote $f_{nj}$ and $F_{nj}$ the ch.f. and cumulative distribution functions of 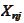$X_{nj}$ respectively.

We think of sums MATH as quantities of similar magnitude even though the number of terms in these sums increases. We formalize such view in the following definition.

Definition

(Holospoudic) The family MATH MATH is called "holospoudic" if the following condition holds MATH

Proposition

(Holospoudic criteria). The family MATH MATH is holospoudic iff MATH

Proof

First, we assume that MATH is true and proceed to prove the statement MATH .

We estimate MATH The last term tends to zero under the assumption MATH and MATH .

Next, we assume that MATH and prove MATH .

We invoke the proposition ( P.m. vs ch.f. estimate ): MATH where $2A=\varepsilon$ , MATH : MATH or MATH The desired statement follows from the above by the proposition ( Dominated convergence theorem ).




A. Lyapunov central limit theorem.
B. Lindeberg-Feller central limit theorem.

Notation. Index. Contents.


















Copyright 2007