his section summarizes the
derivations so far. This is not the only recipe for construction of an MRA.
See the proposition
(
Existence of
smooth compactly supported wavelets
) for a more definitive procedure.
Start from a function
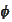with compact support.
Form
,
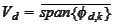closure is in
.
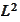The verification of (
Multiresolution
analysis
)-1 should be direct.
For verification of (
Multiresolution
analysis
)-2 use the technique of the proposition
(
Main property of Haar functions
1
) or technique of the section
(
Piecewise linear MRA
).
For verification of (
Multiresolution
analysis
)-3 use the technique illustrated in the section
(
Piecewise linear MRA section
).
The property (
Multiresolution
analysis
)-4 is automatic.
Verify the condition (
Riesz basis
condition
) using the proposition
(
Shifted Fourier transform
equality
) and achieve the orthogonality part of
(
Multiresolution analysis
)-5 by
switching
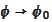according to the
recipe
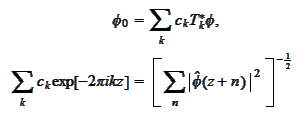of the proposition (
OST property 3
).
|
