uppose the economy has the two risky assets
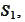
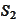 given by the
SDEs
given by the
SDEs
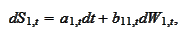
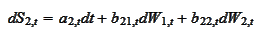 and the MMA
and the MMA
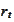 .
The
.
The
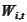 are standard Brownian motions. Similarly to the previous section we again
assume that
are standard Brownian motions. Similarly to the previous section we again
assume that
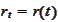 is deterministic and
is deterministic and
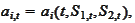
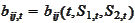 .
Therefore, the price of the derivative
.
Therefore, the price of the derivative
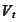 with the payoff
with the payoff
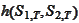 at
at
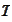 has the functional form
has the functional form
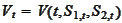 .
We construct the portfolio
.
We construct the portfolio

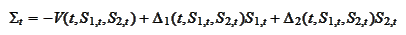 and compute the
increment
and compute the
increment

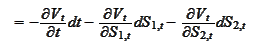
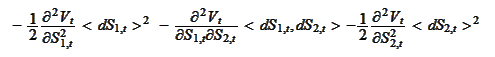
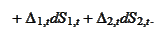 Similarly to the previous section, set
Similarly to the previous section, set
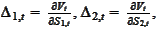 then
then

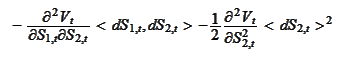 or
or
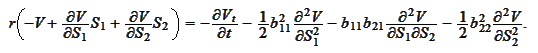 We introduce the function
We introduce the function
 :
:
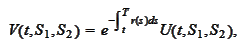 then
then
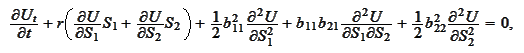
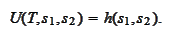 We compare the last result with the proposition
(
Multidimensional
backward Kolmogorov equation
) and
conclude
We compare the last result with the proposition
(
Multidimensional
backward Kolmogorov equation
) and
conclude
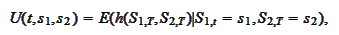
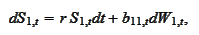
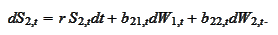
|