e introduce the remainder
Definition
(Remainder of averaged
Taylor
polynomial)
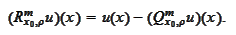
According to the proposition
(
Properties of averaged
Taylor polynomial
)-2 and formula
(
Mollifier for a ball 2
), for a
function
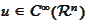 we
have
we
have
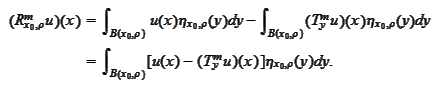 We introduce the function
We introduce the function
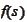 :
:
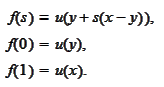 According to the proposition
(
Integral form of Taylor
decomposition
),
According to the proposition
(
Integral form of Taylor
decomposition
),
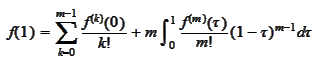 and
and
 Consequently,
Consequently,
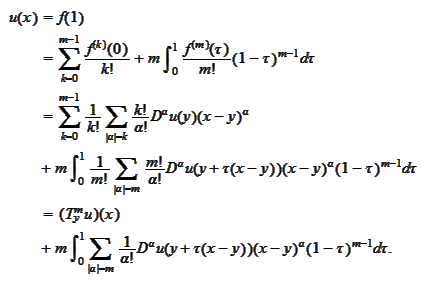
We continue calculation of
 :
:
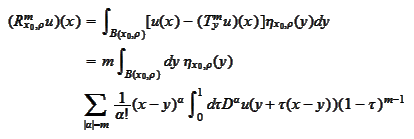 Our goal is to transform the integral to the form
Our goal is to transform the integral to the form
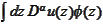 .We
make the change
.We
make the change
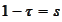 in the
in the
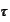 -integral,
-integral,
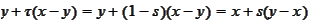 ,
,
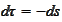 ,
,
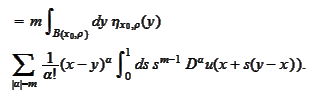 We aim to make a change of variables
We aim to make a change of variables
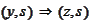 where
where
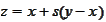 .
Hence, we change the order of integration (see
(
Fubini
theorem
))
.
Hence, we change the order of integration (see
(
Fubini
theorem
))
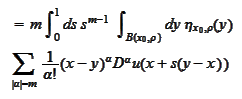 and proceed with the change
and proceed with the change
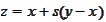 ,
,
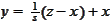 ,
,
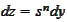 ,
,
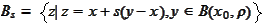
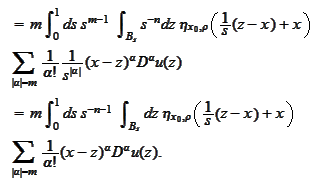 We would like to put result in the form
We would like to put result in the form
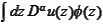 ,
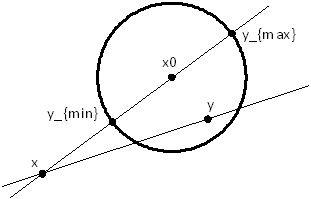
hence, we reverse the order of integration again. The set of integration is
,
hence, we reverse the order of integration again. The set of integration is
 Note that the line
Note that the line
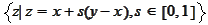 for a fixed
for a fixed
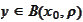 connects
connects
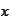 with a point
with a point
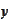 in
in
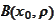 .
Thus, the
.
Thus, the
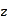 -plane
projection of the set
-plane
projection of the set
 is the convex hull of
is the convex hull of
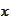 and
and
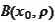 :
:
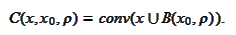 Let
Let
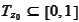 be the section of
be the section of
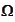 with a plane
with a plane
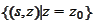 :
:
 We continue the
calculation:
We continue the
calculation:
 where the functions
where the functions
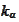 are given
by
are given
by
 We estimate (see the figure
(
Estimation of averaged
Taylor
polynomial
))
We estimate (see the figure
(
Estimation of averaged
Taylor
polynomial
))
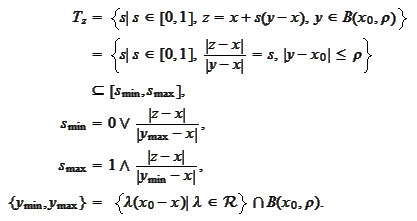
|
Estimation of averaged Taylor
polynomial
|
Thus
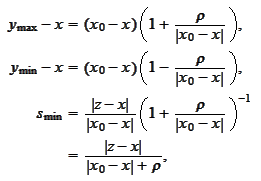 We
state
We
state
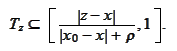 and estimate the
and estimate the
 :
:
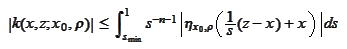 Note that
Note that
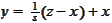 ,
,
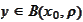 hence
hence
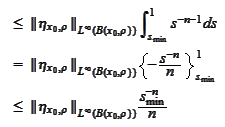 By the formulas (
Mollifier for a ball
1
),(
Mollifier for a ball 2
) and the
definition (
Standard mollifier
definition
)-2
By the formulas (
Mollifier for a ball
1
),(
Mollifier for a ball 2
) and the
definition (
Standard mollifier
definition
)-2
 where the
where the
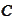 depends on
depends on
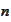 ,
so we drop
,
so we drop
 and substitute for
and substitute for
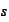 :
:
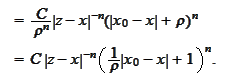 We summarize our findings in the following proposition.
We summarize our findings in the following proposition.
|