e prove the existence part of the proposition
(
Existence and
uniqueness for evolutionary problem
). We follows the path of the section
(
Proof of
existence for stationary problem
) using the proposition
(
A priory estimate for
penalized solution 1
) as the principal tool.
According to the proposition
(
A priory estimate for
penalized solution 1
) and
(
Weak compactness of bounded
set
) we have (along some sequence of values
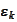 )
)
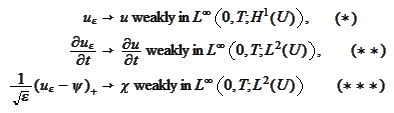 for some
for some
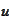 and
and
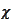 . From
. From
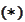 and
and
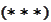 we obtain
we obtain

We complete the proof almost exactly as in the section
(
Proof of
existence for stationary problem
).
|