he
credit default swap contract is designed to provide protection from a credit
event associated with a risky bond. The coupon leg of the CDS pays coupon
while there is no credit event. The protection leg of the credit default swap
pays only if there is a credit event before maturity of the CDS. At the time
of the credit event the protection buyer (=coupon payer) receives par from the
protection seller (=coupon receiver) and delivers the bond to the protection
seller. On default the coupon leg pays the accrued interest.
Let
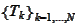 be the coupon payment dates,
be the coupon payment dates,
 be the effective date of the swap,
be the effective date of the swap,
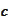 be
the coupon,
be
the coupon,
 the default time (first and the only one),
the default time (first and the only one),
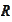 be recovery rate at default and
be recovery rate at default and
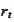 is the MMA interest rate. The value of the coupon leg at time
is the MMA interest rate. The value of the coupon leg at time
 is
is
 The value of the protection leg
is
The value of the protection leg
is
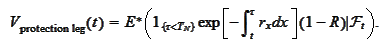 We proceed to evaluate each of the components. We apply the formula
(
Common_application_of_change_of_measure
)
and results of the section
(
T-forward probability
measure
):
We proceed to evaluate each of the components. We apply the formula
(
Common_application_of_change_of_measure
)
and results of the section
(
T-forward probability
measure
):
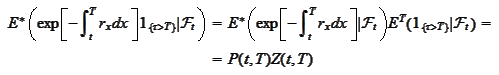 where we introduced the
notation
where we introduced the
notation
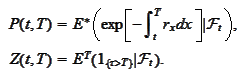 We introduce a fine mesh
We introduce a fine mesh
 on the interval
on the interval
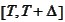 and use the previously developed techniques (see section
(
Distribution of Poisson
process
section
)):
and use the previously developed techniques (see section
(
Distribution of Poisson
process
section
)):
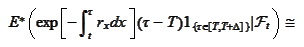
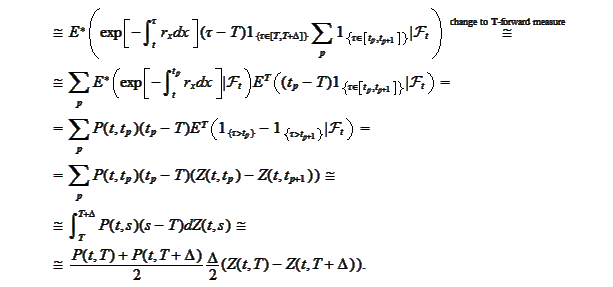 The other leg of the contract is valued in the similar manner:
The other leg of the contract is valued in the similar manner:

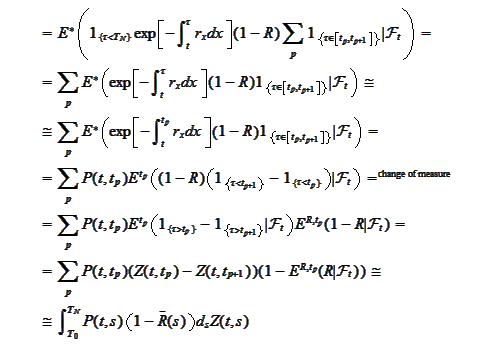 where we introduced the new function
where we introduced the new function
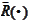 modelling the expectation of the recovery rate. We collect all the pieces
together:
modelling the expectation of the recovery rate. We collect all the pieces
together:
 Conventionally, it is assumed that the
Conventionally, it is assumed that the
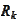 is flat:
is flat:
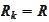 .
.
|