Proof
Let
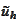 be a solution of the problem
(
Partially
inverted semi discrete parabolic problem 1
) with
be a solution of the problem
(
Partially
inverted semi discrete parabolic problem 1
) with
 .
Then
.
Then
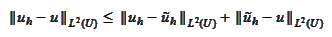 and, for
and, for
 being the solution operator of the problem
(
Partially
inverted semi discrete parabolic problem 1
),
being the solution operator of the problem
(
Partially
inverted semi discrete parabolic problem 1
),
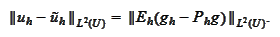 It may be shown that there is an estimate for
It may be shown that there is an estimate for
 similar to the one of the proposition
(
Instant
smoothness of solution operator for PP 1
),
hence
similar to the one of the proposition
(
Instant
smoothness of solution operator for PP 1
),
hence
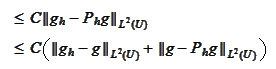 by the conditions of this proposition, the condition
(
Finite dimensional
approximation 1
) and proposition
(
Characterization of H-tilde
spaces
)
by the conditions of this proposition, the condition
(
Finite dimensional
approximation 1
) and proposition
(
Characterization of H-tilde
spaces
)
 Hence, it is enough to prove the statement for
Hence, it is enough to prove the statement for
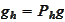 .
.
We intend to apply the proposition
(
Partial inversion lemma 2
)-3. We
introduce
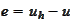 and recall from the proof of the proposition
(
Galerkin convergence 4
)
that
and recall from the proof of the proposition
(
Galerkin convergence 4
)
that
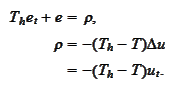 Hence,
Hence,
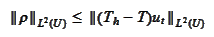 according to the condition
(
Properties of solution
operator
)-2
according to the condition
(
Properties of solution
operator
)-2
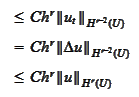 and by the proposition
(
Instant
smoothness of solution operator for PP
1
)
and by the proposition
(
Instant
smoothness of solution operator for PP
1
)
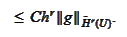 Similarly,
Similarly,
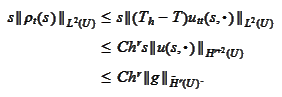 Finally, for any
Finally, for any
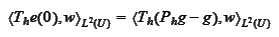 by symmetry of
by symmetry of
 as in the condition (
Properties of
solution
operator
)-1,
as in the condition (
Properties of
solution
operator
)-1,
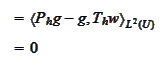 because
because
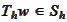 .
Hence,
.
Hence,
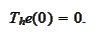 The statement now follows from the proposition
(
Partial inversion lemma 2
)-3.
The statement now follows from the proposition
(
Partial inversion lemma 2
)-3.
|