onsider the
integral
for complex
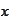and assume that the function
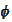is such that
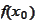exists for some
.
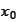Then the integral exists for all
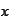s.t.
.
For such
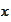we obtain asymptotic expansion for
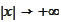via integration by
parts
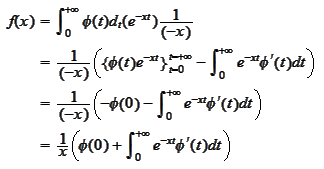and we obtained a combination
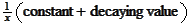as
.
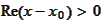We apply the same operation
again:
Thus
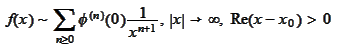where the expansion may be continued as long as
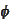is continuously differentiable.
To confirm that the precise values of
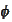for
really are of no relevance to the asymptotic expansion, split the interval of
integration into two
pieces
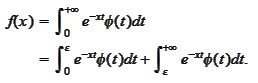for some
.
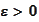Then, for
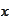s.t.
,
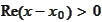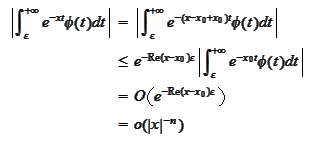for any
.
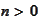We summarize our findings.
|
