Think of
 being an approximation to a hut function (put straddle). The
being an approximation to a hut function (put straddle). The
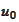 is calculated in the section
(
Decomposition
of payoff function in one dimension
). We place a lot of high level
wavelets around the singularity at the strike. When we evolve
is calculated in the section
(
Decomposition
of payoff function in one dimension
). We place a lot of high level
wavelets around the singularity at the strike. When we evolve
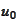 with Backward Kolmogorov's equation, the singularity disappears. Therefore,
the weights
with Backward Kolmogorov's equation, the singularity disappears. Therefore,
the weights
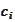 placed on high order wavelets around the strike should redistribute to lower
order wavelets.
placed on high order wavelets around the strike should redistribute to lower
order wavelets.
We
produce
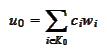 by taking
by taking
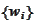 with maximal weights
with maximal weights
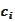 .
Those
.
Those
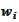 ,
not included in
,
not included in
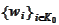 ,
would have a small weight
,
would have a small weight
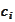 if included in the sum. When we apply the transformation
if included in the sum. When we apply the transformation
 ,
the weights redistribute. The
function
,
the weights redistribute. The
function
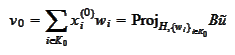 gives us partial information about such redistribution.
gives us partial information about such redistribution.
We form the
set
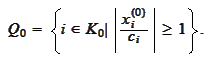
We form the set
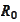 recursively via distinct
unions
recursively via distinct
unions
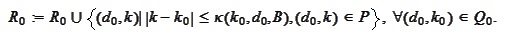 The optimal function
The optimal function
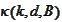 have to depend on diffusive properties of operator
have to depend on diffusive properties of operator
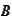 .
However, due to iterative nature of this
procedure,
.
However, due to iterative nature of this
procedure,
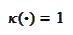 would work too. In addition, we add propagation into higher scales as follows:
would work too. In addition, we add propagation into higher scales as follows:
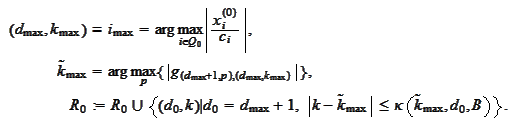 We denote the construction of
We denote the construction of
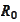 from
from
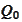 as
as
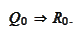
We
calculate
 Then we
calculate
Then we
calculate
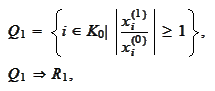
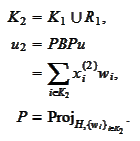 We
continue
We
continue
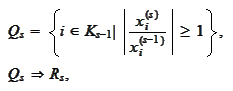
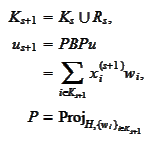 for
for
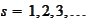 We stop when
We stop when
 becomes small.
becomes small.
After completion, the final set
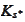 is reduced by removing indexes with lowest contribution. The result is
is reduced by removing indexes with lowest contribution. The result is
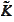 .
.
Another possibility is simply to take
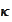 to be a large number and do one iteration.
to be a large number and do one iteration.
|