
|
e
consider a portfolio of several correlated underlyings (obligors)
 .
Each underlying (obligor) pays coupon and may default. A specially created
company (Special Purpose Vehicle) holds such portfolio and issues contracts
called "CDO tranches" that passes both coupons and credit risk on the buyers
of the tranches. We explain below the terms of the contracts.
.
Each underlying (obligor) pays coupon and may default. A specially created
company (Special Purpose Vehicle) holds such portfolio and issues contracts
called "CDO tranches" that passes both coupons and credit risk on the buyers
of the tranches. We explain below the terms of the contracts.
Let
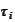 be default time of underlying
be default time of underlying
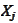 .
Each underlying (=component of the portfolio) is represented by a certain
notional in the portfolio. The fraction of the notional with respect to the
total initial notional is denoted
.
Each underlying (=component of the portfolio) is represented by a certain
notional in the portfolio. The fraction of the notional with respect to the
total initial notional is denoted
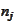 ,
,
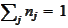 .
We introduce the fractional defaulted
notional
.
We introduce the fractional defaulted
notional
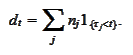 We assume that the recovery at default is the same across the entire
portfolio. We denote such recovery rate by
We assume that the recovery at default is the same across the entire
portfolio. We denote such recovery rate by
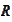 ,
,
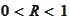 .
Hence, the portfolio loss from the defaults is given
by
.
Hence, the portfolio loss from the defaults is given
by
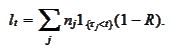 Each tranche is parametrized by "attachment" and "detachment" points that
limit the fractional loss of the tranche
Each tranche is parametrized by "attachment" and "detachment" points that
limit the fractional loss of the tranche
 as
follows
as
follows
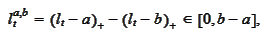
|
|
(Tranche loss)
|
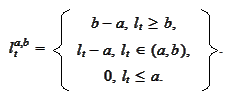 There are several tranches
There are several tranches
 ,
,
 ,
,
 ,
,
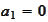 ,
,
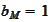 .
The tranche
.
The tranche
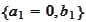 is called "equity" tranche, the tranche
is called "equity" tranche, the tranche
 is called "senior tranche" and the rest of the tranches are called "mezzanine"
tranches. For purposes of calculation of coupon payments we introduce the
notional of the
tranche:
is called "senior tranche" and the rest of the tranches are called "mezzanine"
tranches. For purposes of calculation of coupon payments we introduce the
notional of the
tranche:
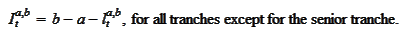 The senior tranche's notional
The senior tranche's notional
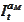 is defined by the
relationship
is defined by the
relationship
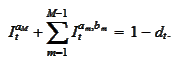
|
|
(Senior tranche loss)
|
The above relationship is necessary to maintain the balance between the coupon
cashflow from the underlying portfolio and the payments to the tranches. For
example, consider the first default in the underlying portfolio. Some notional
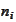 defaults and no longer generates coupon. According to the formula
(
Tranche loss
) the equity tranche covers the loss
defaults and no longer generates coupon. According to the formula
(
Tranche loss
) the equity tranche covers the loss
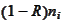 and the notional of the equity tranche decreases by
and the notional of the equity tranche decreases by
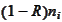 .
However, the entire portfolio's notional decreases by
.
However, the entire portfolio's notional decreases by
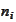 .
Hence, the senior tranche is equipped with the rule that tranche's notional
decreases by
.
Hence, the senior tranche is equipped with the rule that tranche's notional
decreases by
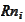 when the equity and mezzanine tranches are taking losses. We confirm that the
formula (
Senior tranche loss
) indeed has
such effect with the following
calculation:
when the equity and mezzanine tranches are taking losses. We confirm that the
formula (
Senior tranche loss
) indeed has
such effect with the following
calculation:
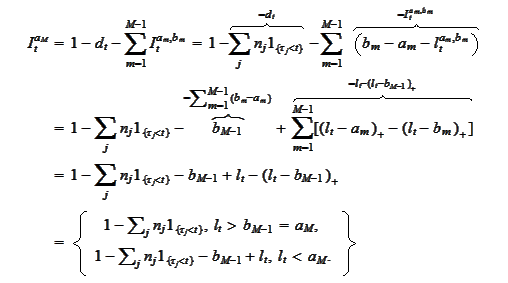 The
The
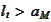 represents the situation when the senior tranche is the only remaining tranche
and it covers the entire loss. The
represents the situation when the senior tranche is the only remaining tranche
and it covers the entire loss. The
 case allows further
transformation
case allows further
transformation
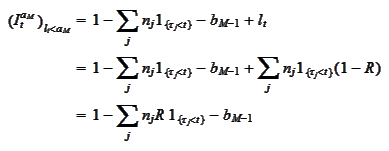 as claimed.
as claimed.
|